Two Equations to Address

The mathematical foundation of the Big Bang model is grounded in Einstein's general theory of relativity (TOR). Since its introduction over a century ago, it has been validated through numerous successful tests, making its rejection improbable. The theory generalizes Newtonian mechanics, accurately depicting the conditions under which the latter fails (such as in strong gravitational fields or near-light-speed motion).

While the fundamental equation of TOR might appear straightforward, it is deceptively simplistic; in practice, it unfolds into ten equations that can only be solved with advanced computational power. Modeling the entire cosmos, with its planets, stars, and galaxies, would be a daunting endeavor. However, the universe's large-scale isotropy and homogeneity simplify this task: at a sufficient distance from our Galaxy, we observe statistically similar cosmic structures.

Galaxies are moving apart in a manner akin to raisins in an expanding dough. If two galaxies are separated by a distance "d," they recede at a speed proportional to that distance. This phenomenon, identified by American astronomer Edwin Hubble in the 1920s, is interpreted in the TOR as the expansion of space, which carries galaxies along with it. The relationship between distance and velocity is described by a value traditionally called the Hubble constant, which, although consistent throughout space, changes over time.

In a homogeneous and isotropic cosmos, only two of the ten TOR equations remain relatively simple and solvable. To reconstruct earlier stages of cosmic evolution, scientists must determine the current density of the cosmic substrate and ascertain the present value of the Hubble constant. Various methods have been employed over decades to achieve increasingly precise measurements.

Infinitely Many Solutions

Now, let’s input our two equations along with the established boundary conditions into a computer (even a standard laptop is sufficient for this modeling). We can then reverse-engineer time according to the TOR.

After less than 14 billion years, we encounter the so-called singularity, where the substrate's density becomes infinitely high. However, this does not signify the universe's inception but rather a warning that we have surpassed the limits of Einstein's theory. Just prior to the singularity, all known physical laws, including TOR, collapse. Strikingly, this transition occurs just a fraction of a nanosecond before the singularity, a time when the universe was filled with quark-gluon plasma, which scientists are studying today using powerful accelerators.

To trace the journey back to the present, we need to establish the initial conditions from the quark-gluon phase. Although these conditions are known from our calculations, we won't use them directly. Instead, we let the computer compute various cosmic evolution scenarios based on randomly selected initial conditions until it replicates the universe we observe today.

Unfortunately, achieving a satisfactory outcome may prove challenging. To align precisely, one must select the substrate density and Hubble constant such that their combination (the so-called ? parameter) equals 1 to twenty-four decimal places.

A value of ? = 1 indicates a flat, indefinitely expanding universe that allows for the formation of galaxies, stars, and planets. Any value below 1 suggests an open universe that expands rapidly, dissolving into near nothingness. Conversely, any value greater than 1 points to a closed universe that eventually collapses back into singularity. Both scenarios can be modeled infinitely, yet the Big Bang theory does not clarify why our universe appears so impeccably flat.

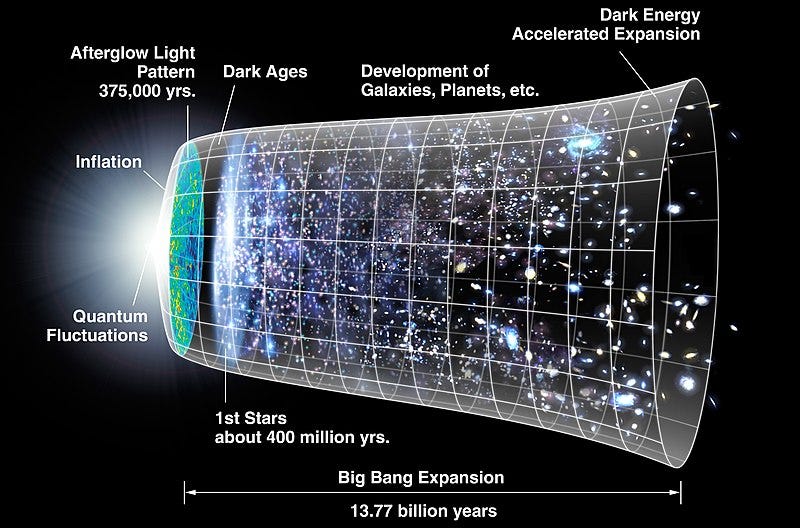
The Mechanism of Rapid Expansion

As time progressed, the cosmic substrate's density and temperature diminished, leading to a series of transformations. The quark-gluon plasma evolved into protons and neutrons, some of which eventually formed the nuclei of deuterium, helium, and lithium. When the cosmos reached an age of 380,000 years, electrons combined with atomic nuclei, releasing light that we now detect as microwave background radiation. This radiation provides a glimpse into the conditions of that early era, revealing that matter was nearly uniformly distributed throughout the universe.

Thus, the cosmos achieved a state of equilibrium, even though the Big Bang theory posits that it should not have had the time to do so. For equilibrium to develop, regions must interact over a significant duration. Observing any two segments of the microwave sky, separated by a few degrees, reveals disconnected areas from the early universe that share the same average density and temperature. Notably, during the 380,000 years of the universe's existence, these regions could not have communicated, even at light speed. Furthermore, tiny variations in density and temperature around average values exhibit identical statistical characteristics across both segments.

A mechanism capable of achieving ideal flattening and homogenization of the cosmos in its nascent stages was proposed by Alan Guth, Andrei Linde, and Alexei Starobinski. Their inflation hypothesis, developed in the late 1970s and early 1980s, suggests a rapid acceleration of cosmic expansion before the quark-gluon epoch. During this inflation, the universe expanded so dramatically that distances increased by a factor of 10^26 (a grain of sand expanded to several million light-years). Consequently, the observer at that time lost sight of the vast majority of the cosmic substrate they initially perceived.

Escape Beyond the Horizon

This process can be elucidated by relating the boundary of the observable universe to the Hubble horizon, beyond which objects recede from the observer faster than the speed of light. Although such movements are prohibited by Einstein's special theory of relativity, this restriction only applies to objects moving through space. In this scenario, we observe the expansion of space itself, which can occur at any speed (similar to how water in a river can flow faster than the fish swimming in it). The horizon is not static; it shifts closer or farther from the observer based on the Hubble constant. During the unique inflationary epoch, it maintained a fixed size, allowing the expanding space to carry objects beyond it.

For the sake of clarity, it can be stated that anything beyond this horizon remains unreachable for study. Following inflation, only a minuscule section of the cosmos remains accessible, devoid of any remnants of the original curvature (an analogy would be a wedding ring resting on an inflated balloon: once inflated, the curvature within the ring becomes imperceptible).

Post-inflation, the universe continues to expand, but at a significantly slower rate, in alignment with the Big Bang theory. The Hubble constant diminishes, causing the horizon to retreat and include portions of the substrate that, according to this theory, never interacted. However, they appear statistically similar because they were in contact before inflation and became disconnected during the inflationary phase.

Inflation also addresses the origin of the minute yet critically significant density fluctuations in the early universe, which developed into the cosmic structures we observe today, from superclusters to galaxies, stars, and planetary systems. These fluctuations stem from self-excited quantum variations of the field associated with inflation. The nature of this field, termed the inflaton field, remains unknown, but the consistency between predicted fluctuations and observed data lends considerable weight to the inflation hypothesis.

The First Cry of the Universe

Critics of inflation note the steep costs associated with resolving the challenges posed by the Big Bang theory. By fine-tuning the parameters of the inflaton field, one can generate various post-inflationary universes, of which only a select few align with empirical observations. Instead of eliminating problems, they often seem to be merely shifted elsewhere. Furthermore, this theory requires us to accept that a significant portion of the cosmos is entirely beyond our reach. The scale of this inaccessible region is uncertain, but it is undoubtedly much larger than our observable horizon.

Even more concerning is the possibility of eternal inflation, leading to a multitude of unreachable universes, commonly referred to as the multiverse. Paul Steinhardt, a prominent cosmologist at Princeton University, was so disheartened by these implications that he transitioned from supporting the inflation hypothesis to opposing it, proposing an alternative concept known as the cyclic universe.

Nonetheless, the debates among cosmologists are not entirely futile, as differing perspectives on the early universe yield varying predictions that can be empirically tested. Specifically, researchers are focused on the gravitational waves generated in the first picoseconds of the universe's existence, which should leave a distinct mark in microwave radiation. Although no such signal has yet been detected, should it emerge with just double the sensitivity of current detectors, inflation would likely be the only viable explanation left standing. Competing theories, which anticipate weaker signals, would be effectively ruled out.

While the early history of the universe remains shrouded in uncertainty, its eventual fate appears more definitive. Discovered a quarter-century ago, dark energy plays a role similar to that of the inflaton field: it accelerates cosmic expansion. Presently, this acceleration is gradual, but if observations are interpreted accurately, the universe may eventually expand to such an extent that it will "leak" from our observable horizon. Future observers may perceive only the Milky Way and a handful of its closest neighbors, with all other galaxies slipping beyond their reach.

Did you enjoy this exploration of cosmic inflation? If so, please consider leaving a comment or some applause to encourage more engaging content. Follow for daily articles and updates. Thank you!